
- Discrete Mathematics Tutorial
- Discrete Mathematics - Home
- Discrete Mathematics Introduction
- Mathematical Statements and Operations
- Atomic and Molecular Statements
- Implications
- Predicates and Quantifiers
- Sets, Relations, & Functions
- Sets
- Sets and Notations
- Relations
- Operations on Sets
- Venn Diagrams on Sets
- Functions
- Surjection and Bijection Functions
- Image and Inverse-Image
- Mathematical Logic
- Propositional Logic
- Logical Equivalence
- Deductions
- Predicate Logic
- Proof by Contrapositive
- Proof by Contradiction
- Proof by Cases
- Rules of Inference
- Group Theory
- Operators & Postulates
- Group Theory
- Algebric Structure for Groups
- Abelian Group
- Semi Group
- Monoid
- Rings and Subring
- Properties of Rings
- Integral Domain
- Fields
- Counting & Probability
- Counting Theory
- Combinatorics
- Additive and Multiplicative Principles
- Counting with Sets
- Inclusion and Exclusion
- Bit Strings
- Lattice Path
- Binomial Coefficients
- Pascal's Triangle
- Permutations and Combinations
- Pigeonhole Principle
- Probability Theory
- Probability
- Sample Space, Outcomes, Events
- Conditional Probability and Independence
- Random Variables in Probability Theory
- Distribution Functions in Probability Theory
- Variance and Standard Deviation
- Mathematical & Recurrence
- Mathematical Induction
- Formalizing Proofs for Mathematical Induction
- Strong and Weak Induction
- Recurrence Relation
- Linear Recurrence Relations
- Non-Homogeneous Recurrence Relations
- Solving Recurrence Relations
- Master's Theorem
- Generating Functions
- Graph Theory
- Graph & Graph Models
- More on Graphs
- Planar Graphs
- Non-Planar Graphs
- Polyhedra
- Introduction to Trees
- Properties of Trees
- Rooted and Unrooted Trees
- Spanning Trees
- Graph Coloring
- Coloring Theory in General
- Coloring Edges
- Euler Paths and Circuits
- Hamiltonion Path
- Boolean Algebra
- Boolean Expressions & Functions
- Simplification of Boolean Functions
- Advanced Topics
- Number Theory
- Divisibility
- Remainder Classes
- Properties of Congruence
- Solving Linear Diophantine Equation
- Useful Resources
- Quick Guide
- Useful Resources
- Discussion
Introduction to Trees
Tree is a discrete structure that represents hierarchical relationships between individual elements or nodes. A tree in which a parent has no more than two children is called a binary tree.
Tree and its Properties
Definition − A Tree is a connected acyclic undirected graph. There is a unique path between every pair of vertices in G. A tree with N number of vertices contains (N−1) number of edges. The vertex which is of 0 degree is called root of the tree. The vertex which is of 1 degree is called leaf node of the tree and the degree of an internal node is at least 2.
Example − The following is an example of a tree −
Centers and Bi-Centers of a Tree
The center of a tree is a vertex with minimal eccentricity. The eccentricity of a vertex X in a tree G is the maximum distance between the vertex X and any other vertex of the tree. The maximum eccentricity is the tree diameter. If a tree has only one center, it is called Central Tree and if a tree has only more than one centers, it is called Bi-central Tree. Every tree is either central or bi-central.
Algorithm to find centers and bi-centers of a tree
Step 1 − Remove all the vertices of degree 1 from the given tree and also remove their incident edges.
Step 2 − Repeat step 1 until either a single vertex or two vertices joined by an edge is left. If a single vertex is left then it is the center of the tree and if two vertices joined by an edge is left then it is the bi-center of the tree.
Problem 1
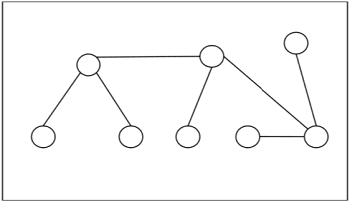
Find out the center/bi-center of the following tree −

Solution
At first, we will remove all vertices of degree 1 and also remove their incident edges and get the following tree −
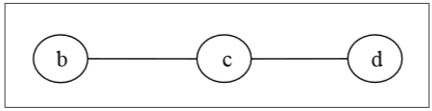
Again, we will remove all vertices of degree 1 and also remove their incident edges and get the following tree −
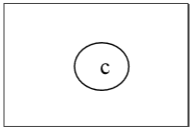
Finally we got a single vertex c and we stop the algorithm. As there is single vertex, this tree has one center c and the tree is a central tree.
Problem 2
Find out the center/bi-center of the following tree −
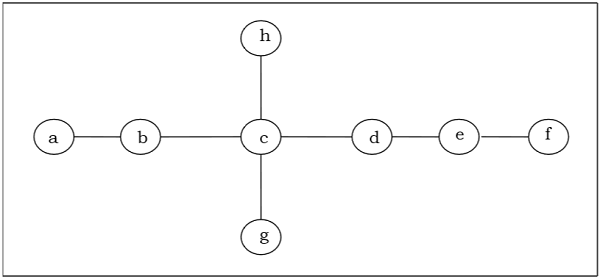
Solution
At first, we will remove all vertices of degree 1 and also remove their incident edges and get the following tree −

Again, we will remove all vertices of degree 1 and also remove their incident edges and get the following tree −
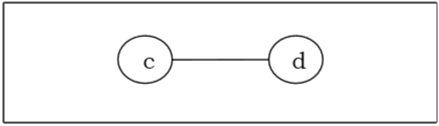
Finally, we got two vertices c and d left, hence we stop the algorithm. As two vertices joined by an edge is left, this tree has bi-center cd and the tree is bi-central.
Labeled Trees
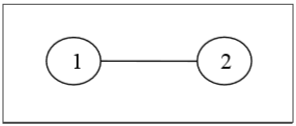
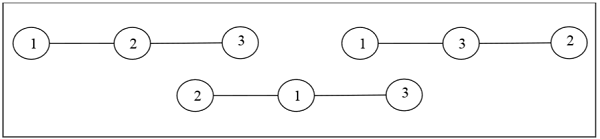
Definition − A labeled tree is a tree the vertices of which are assigned unique numbers from 1 to n. We can count such trees for small values of n by hand so as to conjecture a general formula. The number of labeled trees of n number of vertices is nn−2. Two labeled trees are isomorphic if their graphs are isomorphic and the corresponding points of the two trees have the same labels.
Example
Unlabeled Trees
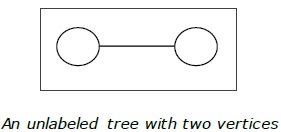
Definition − An unlabeled tree is a tree the vertices of which are not assigned any numbers. The number of labeled trees of n number of vertices is (2n)!(n+1)!n! (nth Catalan number)
Example
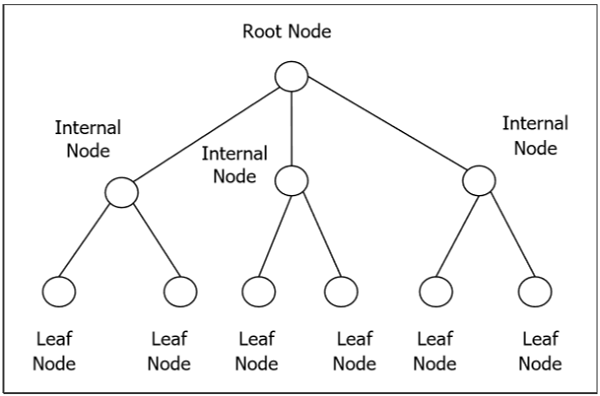
Rooted Tree
A rooted tree G is a connected acyclic graph with a special node that is called the root of the tree and every edge directly or indirectly originates from the root. An ordered rooted tree is a rooted tree where the children of each internal vertex are ordered. If every internal vertex of a rooted tree has not more than m children, it is called an m-ary tree. If every internal vertex of a rooted tree has exactly m children, it is called a full m-ary tree. If m=2, the rooted tree is called a binary tree.
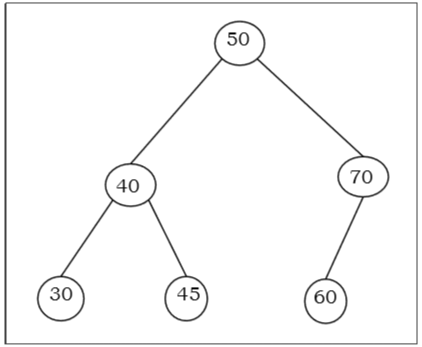
Binary Search Tree
Binary Search tree is a binary tree which satisfies the following property −
- X in left sub-tree of vertex V,Value(X)≤Value(V)
- Y in right sub-tree of vertex V,Value(Y)≥Value(V)
So, the value of all the vertices of the left sub-tree of an internal node V are less than or equal to V and the value of all the vertices of the right sub-tree of the internal node V are greater than or equal to V. The number of links from the root node to the deepest node is the height of the Binary Search Tree.
Example
Algorithm to search for a key in BST
BST_Search(x, k) if ( x = NIL or k = Value[x] ) return x; if ( k < Value[x]) return BST_Search (left[x], k); else return BST_Search (right[x], k)
Complexity of Binary search tree
| Average Case | Worst case | |
|---|---|---|
| Space Complexity | O(n) | O(n) |
| Search Complexity | O(log n) | O(n) |
| Insertion Complexity | O(log n) | O(n) |
| Deletion Complexity | O(log n) | O(n) |