
- Automata Theory Tutorial
- Automata Theory - Home
- Automata Theory - Getting Started
- Automata Theory - History
- Automata Theory - Applications
- Automata Terminology
- Basics of String in Automata
- Set Theory for Automata
- Finite Sets and Infinite Sets
- Algebraic Operations on Sets
- Relations Sets in Automata Theory
- Graph and Tree in Automata Theory
- Transition Table in Automata
- What is Queue Automata?
- Compound Finite Automata
- Complementation Process in DFA
- Closure Properties in Automata
- Concatenation Process in DFA
- Language and Grammars
- Language and Grammar
- Grammars in Theory of Computation
- Language Generated by a Grammar
- Chomsky Classification of Grammars
- Context-Sensitive Languages
- Finite Automata
- What is Finite Automata?
- Finite Automata Types
- Applications of Finite Automata
- Limitations of Finite Automata
- Two-way Deterministic Finite Automata
- Deterministic Finite Automaton (DFA)
- Non-deterministic Finite Automaton (NFA)
- NDFA to DFA Conversion
- Equivalence of NFA and DFA
- Dead State in Finite Automata
- Minimization of DFA
- Automata Moore Machine
- Automata Mealy Machine
- Moore vs Mealy Machines
- Moore to Mealy Machine
- Mealy to Moore Machine
- Myhill–Nerode Theorem
- Mealy Machine for 1’s Complement
- Finite Automata Exercises
- Complement of DFA
- Regular Expressions
- Regular Expression in Automata
- Regular Expression Identities
- Applications of Regular Expression
- Regular Expressions vs Regular Grammar
- Kleene Closure in Automata
- Arden’s Theorem in Automata
- Convert Regular Expression to Finite Automata
- Conversion of Regular Expression to DFA
- Equivalence of Two Finite Automata
- Equivalence of Two Regular Expressions
- Convert Regular Expression to Regular Grammar
- Convert Regular Grammar to Finite Automata
- Pumping Lemma in Theory of Computation
- Pumping Lemma for Regular Grammar
- Pumping Lemma for Regular Expression
- Pumping Lemma for Regular Languages
- Applications of Pumping Lemma
- Closure Properties of Regular Set
- Closure Properties of Regular Language
- Decision Problems for Regular Languages
- Decision Problems for Automata and Grammars
- Conversion of Epsilon-NFA to DFA
- Regular Sets in Theory of Computation
- Context-Free Grammars
- Context-Free Grammars (CFG)
- Derivation Tree
- Parse Tree
- Ambiguity in Context-Free Grammar
- CFG vs Regular Grammar
- Applications of Context-Free Grammar
- Left Recursion and Left Factoring
- Closure Properties of Context Free Languages
- Simplifying Context Free Grammars
- Removal of Useless Symbols in CFG
- Removal Unit Production in CFG
- Removal of Null Productions in CFG
- Linear Grammar
- Chomsky Normal Form (CNF)
- Greibach Normal Form (GNF)
- Pumping Lemma for Context-Free Grammars
- Decision Problems of CFG
- Pushdown Automata
- Pushdown Automata (PDA)
- Pushdown Automata Acceptance
- Deterministic Pushdown Automata
- Non-deterministic Pushdown Automata
- Construction of PDA from CFG
- CFG Equivalent to PDA Conversion
- Pushdown Automata Graphical Notation
- Pushdown Automata and Parsing
- Two-stack Pushdown Automata
- Turing Machines
- Basics of Turing Machine (TM)
- Representation of Turing Machine
- Examples of Turing Machine
- Turing Machine Accepted Languages
- Variations of Turing Machine
- Multi-tape Turing Machine
- Multi-head Turing Machine
- Multitrack Turing Machine
- Non-Deterministic Turing Machine
- Semi-Infinite Tape Turing Machine
- K-dimensional Turing Machine
- Enumerator Turing Machine
- Universal Turing Machine
- Restricted Turing Machine
- Convert Regular Expression to Turing Machine
- Two-stack PDA and Turing Machine
- Turing Machine as Integer Function
- Post–Turing Machine
- Turing Machine for Addition
- Turing Machine for Copying Data
- Turing Machine as Comparator
- Turing Machine for Multiplication
- Turing Machine for Subtraction
- Modifications to Standard Turing Machine
- Linear-Bounded Automata (LBA)
- Church's Thesis for Turing Machine
- Recursively Enumerable Language
- Computability & Undecidability
- Turing Language Decidability
- Undecidable Languages
- Turing Machine and Grammar
- Kuroda Normal Form
- Converting Grammar to Kuroda Normal Form
- Decidability
- Undecidability
- Reducibility
- Halting Problem
- Turing Machine Halting Problem
- Rice's Theorem in Theory of Computation
- Post’s Correspondence Problem (PCP)
- Types of Functions
- Recursive Functions
- Injective Functions
- Surjective Function
- Bijective Function
- Partial Recursive Function
- Total Recursive Function
- Primitive Recursive Function
- μ Recursive Function
- Ackermann’s Function
- Russell’s Paradox
- Gödel Numbering
- Recursive Enumerations
- Kleene's Theorem
- Kleene's Recursion Theorem
- Advanced Concepts
- Matrix Grammars
- Probabilistic Finite Automata
- Cellular Automata
- Reduction of CFG
- Reduction Theorem
- Regular expression to ∈-NFA
- Quotient Operation
- Parikh’s Theorem
- Ladner’s Theorem
- Automata Theory Resources
- Automata - Quick Guide
- Automata - Resources
- Automata - Discussion
Non-deterministic Finite Automaton
In NDFA, for a particular input symbol, the machine can move to any combination of the states in the machine. In other words, the exact state to which the machine moves cannot be determined. Hence, it is called Non-deterministic Automaton. As it has finite number of states, the machine is called Non-deterministic Finite Machine or Non-deterministic Finite Automaton.
Formal Definition of an NDFA
An NDFA can be represented by a 5-tuple (Q, ∑, δ, q0, F) where −
Q is a finite set of states.
∑ is a finite set of symbols called the alphabets.
δ is the transition function where δ: Q × ∑ → 2Q
(Here the power set of Q (2Q) has been taken because in case of NDFA, from a state, transition can occur to any combination of Q states)
q0 is the initial state from where any input is processed (q0 ∈ Q).
F is a set of final state/states of Q (F ⊆ Q).
Graphical Representation of an NDFA: (same as DFA)
An NDFA is represented by digraphs called state diagram.
- The vertices represent the states.
- The arcs labeled with an input alphabet show the transitions.
- The initial state is denoted by an empty single incoming arc.
- The final state is indicated by double circles.
Example
Let a non-deterministic finite automaton be →
- Q = {a, b, c}
- ∑ = {0, 1}
- q0 = {a}
- F = {c}
The transition function δ as shown below −
| Present State | Next State for Input 0 | Next State for Input 1 |
|---|---|---|
| a | a, b | b |
| b | c | a, c |
| c | b, c | c |
Its graphical representation would be as follows −

DFA vs NDFA
The following table lists the differences between DFA and NDFA.
| DFA | NDFA |
|---|---|
| The transition from a state is to a single particular next state for each input symbol. Hence it is called deterministic. | The transition from a state can be to multiple next states for each input symbol. Hence it is called non-deterministic. |
| Empty string transitions are not seen in DFA. | NDFA permits empty string transitions. |
| Backtracking is allowed in DFA | In NDFA, backtracking is not always possible. |
| Requires more space. | Requires less space. |
| A string is accepted by a DFA, if it transits to a final state. | A string is accepted by a NDFA, if at least one of all possible transitions ends in a final state. |
Acceptors, Classifiers, and Transducers
Acceptor (Recognizer)
An automaton that computes a Boolean function is called an acceptor. All the states of an acceptor is either accepting or rejecting the inputs given to it.
Classifier
A classifier has more than two final states and it gives a single output when it terminates.
Transducer
An automaton that produces outputs based on current input and/or previous state is called a transducer. Transducers can be of two types −
Mealy Machine − The output depends both on the current state and the current input.
Moore Machine − The output depends only on the current state.
Acceptability by DFA and NDFA
A string is accepted by a DFA/NDFA iff the DFA/NDFA starting at the initial state ends in an accepting state (any of the final states) after reading the string wholly.
A string S is accepted by a DFA/NDFA (Q, ∑, δ, q0, F), iff
δ*(q0, S) ∈ F
The language L accepted by DFA/NDFA is
{S | S ∈ ∑* and δ*(q0, S) ∈ F}
A string S is not accepted by a DFA/NDFA (Q, ∑, δ, q0, F), iff
δ*(q0, S) ∉ F
The language L not accepted by DFA/NDFA (Complement of accepted language L) is
{S | S ∈ ∑* and δ*(q0, S) ∉ F}
Example
Let us consider the DFA shown in Figure 1.3. From the DFA, the acceptable strings can be derived.
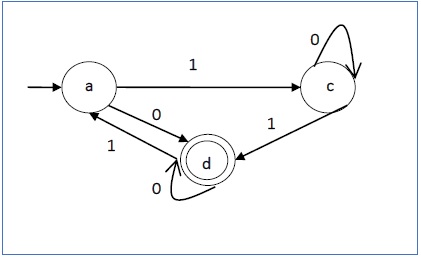
Strings accepted by the above DFA: {0, 00, 11, 010, 101, ...........}
Strings not accepted by the above DFA: {1, 011, 111, ........}